Blog

Introduction

As we have seen earlier in the chapter of Principle of UV-Vis Spectroscopy, absorption of a particular wavelength of light depends upon the π-electron system of the molecule. The more the conjugation of the π-electron system within the molecule, the higher the wavelength of light it can absorb. Robert Burns Woodward and Louis Fieser put down a set of rules which allows one to calculate the wavelength of maximum absorption (λmax) for a molecule empirically. These sets of rules to calculate the wavelength of maximum absorption or λmax of a compound in the ultraviolet-visible spectrum, based empirically have been called the Woodward-Fieser rules or Woodward’s-rules.

These sets of article aims to guide the student on how to use these rules to calculate the wavelength of maximum absorption or λmax for different systems. The following pages are listed for the readers.

1. Conjugated Dienes and Polyenes

2. Conjugated Carbonyl Compounds

3. Sample Problems using Woodward-Fieser Rules

Note: Numerical values for Woodward-Fieser rules differ slightly from one textbook to another. We have tried to compile an extensive list of numerical values from online resources, textbooks and journal articles based on the popularity of the number. It is recommended that you learn on how to apply the values for the contributors and then follow the values given in a text book recommended by your teacher, or use our values. We believe that learning how to apply the rules is more essential than actually getting the exact answer. Other’s opinions may vary.

Woodward-Fieser Rules for Calculating the λmax of Conjugated Carbonyl Compounds

Woodward-Fieser rules can be extended to calculate the λmax of α,β-unsaturated carbonyl compounds. In a similar manner to Woodward rules for dienes discussed previously, there is base value to which the substituent effects can be added and the λmax can be calculated using the formula:

λmax = Base value + Σ Substituent Contributions + Σ Other Contributions

Table 2: Gives the values for the influence of different chromophores in conjugated carbonyl systems as per Woodward-Fieser rules. The usage of these will become more evident in the examples which follow.

Class	Chromophore	Influence to λmax
Core Chromophore Base Value
α,β-unsaturated carbonyl compound	If R = -H (α,β-unsaturated aldehyde) If R = -Alkyl (α,β-unsaturated ketone) If R = -OR (α,β-unsaturated ester)	+ 210 nm + 215 nm + 195 nm
	Cyclopentenone	+ 202 nm
	Cyclohexenone	+ 215 nm
α,β-γ,δ-diene carbonyl compound	If R = -H ( α,β-γ,δ-diene aldehyde) If R = -Alkyl ( α,β-γ,δ-diene ketone) If R = -OR ( α,β-γ,δ-diene ester)	+210 + 30 =+240 nm +215 + 30 = +245 nm +195 + 30 = +225 nm
Substituent Effects at α-position	-R (Alkyl group)	+ 10 nm
	-OR (Alkoxy group)	+ 35 nm
	-Cl (Chloro group)	+ 15 nm
	-Br (Bromo group)	+ 25 nm
	-OH (alcohol/hydroxyl)	+ 35 nm
	-OC(O)R (Acyloxy/Ester)	+  6 nm
Substituent Effects at β-position	-R (Alkyl group)	+ 12 nm
	-OR (Alkoxy group)	+ 30 nm
	-Cl (Chloro group)	+ 12 nm
	-Br (Bromo group)	+ 30 nm
	-OH (alcohol/hydroxyl)	+ 30 nm
	-OC(O)R (Acyloxy/Ester)	+ 6 nm
	-SR (Sulfide)	+ 85 nm
	-NR2 (Amine)	+ 95 nm
Substituent Effects at γ and δ-position	-R (Alkyl group) (both γ and δ)	+ 18 nm
	-OC(O)R (Acyloxy/Ester) (both γ and δ)	+ 6 nm
	-Cl (Chloro) (both γ and δ)	+ 12 nm
	-Br (Bromo) (both γ and δ)	+ 30 nm
	-OH (alcohol/hydroxyl group) (only γ)	+ 50 nm
	-OR (Alkoxy group) (only γ)	+ 30 nm
Further π-conjugation	– C=C (double bond extending conjugation)	+ 30 nm
	– C6H5 (Phenyl group)	+ 60 nm
Other Contributors	Exocyclic Double Bond	+ 5 nm
	Homoannular cyclohexadiene	+ 35 nm
	Solvent Effects
	1] Water	– 8 nm
	2] Methanol/Ethanol	– 1 nm
	3] Ether	+ 6 nm
	4] Hexane / Cyclohexane	+ 7 nm

Let us discuss each of the above values and when to apply them in greater detail with examples:

Core Chromophores With Base Values

As Woodward and Fieser have listed, α,β-unsaturated carbonyl compounds have a range of influence on the λmax of the molecule depending upon:

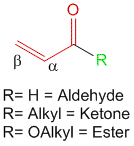
1] The type of carbonyl functionality present. For example, α,β-unsaturated aldehyde contribute 210 nm while α,β-unsaturated ketones contribute 215 nm and α,β-unsaturated esters contribute 195 nm.

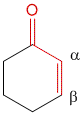
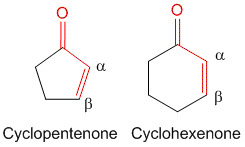
2] If the core is a part of a cyclic ring. For example, cyclopentenone contribution is 202 nm while cyclohexenone is 215 nm.

3] If the conjugation is extended to γ,δ-positions to form dienes. For example, in such cases, a simple addition of 30 nm to the base value of the α,β-unsaturated carbonyl compound gives appropriate estimates to the observed influences.

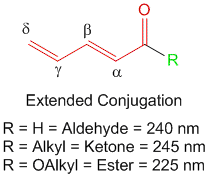
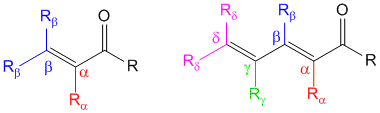
Note- In cases of α,β-γ,δ-diene carbonyl compounds like those shown above, the extended conjugation at the α,β-γ,δ-positions is accounted for in the base value of the core chromophore and need not be added separately. If however there is another substituent at the α,β-γ,δ-positions, then you must add an additional + 30 nm for each. Also the bond shown as β-γ is not counted as β substituent but as a part of the core chromophore and need not be added separately.

Substituent Effects

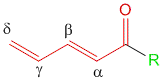
According to Woodward, in case of α,β-unsaturated carbonyl compounds, the location of the substituent is significant in determining the influence on the wavelength of maximum absorption. Substituents can be located on either α, β positions. If the conjugation is extended to γ and δ positions, then substitutions at these position also play a vital role in determining the practical λmax.

1] Substituents at α-Position

As we can see the from table 3 below the effect of different substituent when placed on the α-position.

Table 3: Effect of substituents on the α-position of α,β-unsaturated carbonyl compounds

2] Substituents at β-Position

As we can see the from table 4 below the effect of different substituent when placed on the β-position.

Table 4: Effect of substituents on the β-position of α,β-unsaturated carbonyl compounds

3] Substituents at γ and δ-position

As we can see the from table 5 below the effect of different substituent when placed on the γ or δ position.

Table 5: Effect of substituents on the γ or δ position of α,β-γ,δ-diene carbonyl compound.

Other Contributors

1] Exocyclic Double Bonds

In general exocyclic double bonds add an additional + 5 nm to the base value. In order to identify exocyclic double bonds we recommend you read the previous chapter on how to use Woodward-Fieser rules to calculate the λmax of conjugated dienes and polyenes. We have explained it extensively there.

2] Solvent Effects

Since carbonyl functional groups have polarity, solvents play an important role in how the electronics of the structure play out. The rules are simple and straight forward:

3] Homoannular Cyclohexadiene

In a special case where you have α,β-γ,δ-diene carbonyl compound and both the double bonds are present within one ring system you get a homoannular or homocyclic cyclohexadiene carbonyl compound. In such a case you must add an additional 35 nm to the system.

Sample Problems to use Woodward-Fieser Rules to Calculate λmax of Conjugated Carbonyl Compounds

Check out the page having sample problems using Woodward rules to gain insight on how to calculate the λmax of conjugated carbonyl compounds. Also check out the section on Woodward rules for dienes and polyenes.

Books on Analytical Chemistry and Spectroscopy

Check out these good books for analytical chemistry and spectroscopy

References

1. Woodward, R. B. Structure and Absoprtion Spectra. IV. Further Observations on α,β-Unsaturated Ketones. J. Am. Chem. Soc. 1942. 64(1), 76-77.
2. Woodward, R. B. Clifford, A. F. Structure and Absoprtion Spectra. II. 3-Acetoxy-Δ5-(6)-nor-cholestene-7-carboxylic Acid. J. Am. Chem. Soc. 1941. 63(10), 2727-2729.
3. Spectroscopic Determination of Organic Compounds, 5th Edition, Silverstein, Bassler, Morrill 1991.
4. Kalsi, P. S. Spectroscopy of Organic Compounds. 6th Edition, New Age International Publishers, New Delhi, 2004.
5. Glagovich, N. Woodward’s rules for conjugated carbonyl compounds. [Online]http://www.chemistry.ccsu.edu/glagovich/teaching/316/uvvis/conjugated.html (Accessed on July 27, 2012).
6. Author Unknown. Michigan State University – Department of Chemistry. Woodward-Fieser Rules for Calculating the π to  π* λmax of Conjugated Carbonyl Compounds. [Online]http://www2.chemistry.msu.edu/faculty/reusch/VirtTxtJml/Spectrpy/UV-Vis/uvspec.htm (Accessed on July 27, 2012).